 ;
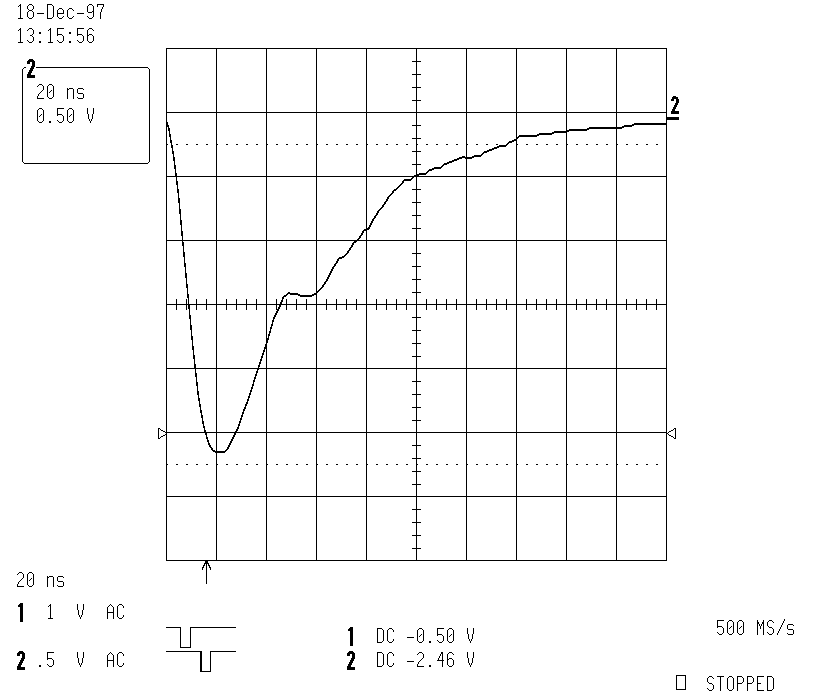
Figure 1. Typical raw PMT pulse.
;
Figure 1. Typical raw PMT pulse.
Cy Hoffman, Rich Miller, Stefan Westerhoff
In order to get a better handle on the scattering and absorption of light in the water, we have attempted to estimate these parameters by using the Igloos currently in the pond. The method relies on the fact that the tyvek is an excellent, diffuse reflector. Light produced in the igloo should bounce around becoming "isotropized" until it is either absorbed in the water (or the tyvek) or strikes the PMT. The idea, then, is to measure the time distribution of the light detected by the PMT; if this model is correct, the distribution after the inital sharp rise should be an exponential with a time constant characteristic of the absorption length in water. The relationship of absorption length (L) to time constant (tau) is:
L = (c/n) * tau
where c is the velocity of light and n is the index of refraction of water.
We took measurements with Jordan's Wonderscope on igloo #7, triggering on two scintillator paddles beneath the igloo. We used David Williams HV/signal pickoff circuit (to remove the long time constant in the front-end board).
The resulting pulses have the expected shape: a sharp rise (~15
ns 10%-90%) and an exponetial tail punctuated by a glitch due to a reflection
(probably due to the transition from 75 ohms to 50 ohms) about 30 ns after
the peak. A typical pulse is shown in figure 1 below.
Alas the scope does not allow a semilog vertical scale so the data had
to be stored and refit by hand. The time-constant of the exponential
is -0.023 +/- 0.001. This translates to an absorption length of 10.4m.
Note that any absorption by the tyvek will serve to decrease the derived
absorption length in water; thus this is a lower limit on the absorption
length in the Igloo water.
 ;
Figure 1. Typical raw PMT pulse.
;
Figure 1. Typical raw PMT pulse.
The derived absorption length is an average over wavelength appropriately weighted by the Cerenkov spectrum, the absorption in water, the reflectivity and absorption in tyvek, and the PMT response. And, of course, this relates to the water in the igloos, not in the rest of the pond. With these caveats, the implication is that the attenuation length that Michael measures is predominantly scattering, not absorption. This agrees with Gus' observation when he went into the pond to replace some PMTs. Finally, this is also in agreement with the observed long tail in the light arrival time in the shower layer, which is significantly larger than expected from the Monte Carlo without light scattering.